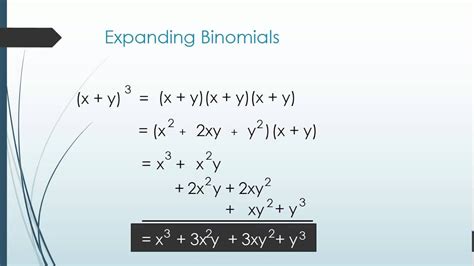
Binomial expansion induction proof
 Series Khan Academy)
WebBinomial functions and Taylor series (Sect. 10.10) I Review: The Taylor Theorem. I The binomial function. I Evaluating non-elementary integrals. I The Euler identity. I Taylor series table. Review: The Taylor Theorem Recall: If f : D → R is infinitely differentiable, and a, x ∈ D, then f (x) = T n(x)+ R n(x), where the Taylor polynomial T n and the Remainder … Inductionyields another proof of the binomial theorem. When n= 0, both sides equal 1, since x0= 1and (00)=1.{\displaystyle {\tbinom {0}{0}}=1.} Now suppose that the equality holds for a given n; we will prove it for n+ 1. For j, k≥ 0, let [f(x, y)]j,kdenote the coefficient of xjykin the polynomial f(x, y). See more In elementary algebra, the binomial theorem (or binomial expansion) describes the algebraic expansion of powers of a binomial. According to the theorem, it is possible to expand the polynomial (x + y) into a See more Special cases of the binomial theorem were known since at least the 4th century BC when Greek mathematician Euclid mentioned the special case of the binomial theorem for … See more The coefficients that appear in the binomial expansion are called binomial coefficients. These are usually written $${\displaystyle {\tbinom {n}{k}},}$$ and pronounced "n choose k". Formulas The coefficient of x … See more • The binomial theorem is mentioned in the Major-General's Song in the comic opera The Pirates of Penzance. • Professor Moriarty is described by Sherlock Holmes as having written See more Here are the first few cases of the binomial theorem: • the exponents of x in the terms are n, n − 1, ..., 2, 1, 0 (the last term implicitly contains x = 1); See more Newton's generalized binomial theorem Around 1665, Isaac Newton generalized the binomial theorem to allow real exponents other than … See more The binomial theorem is valid more generally for two elements x and y in a ring, or even a semiring, provided that xy = yx. For example, it … See more
Binomial expansion induction proof
Did you know?
WebJan 4, 2016 · In this episode we introduce the process of mathematical induction, a powerful tool for proofs. We use this to prove a formula for binomial expansion for all... WebAug 16, 2024 · The binomial theorem gives us a formula for expanding (x + y)n, where n is a nonnegative integer. The coefficients of this expansion are precisely the binomial …
Webwhere is the binomial coefficient and denotes the j th derivative of f (and in particular ). The rule can be proved by using the product rule and mathematical induction . Second derivative [ edit] If, for example, n = 2, the rule gives an expression for the second derivative of a product of two functions: More than two factors [ edit] WebFortunately, the Binomial Theorem gives us the expansion for any positive integer power of (x + y) : For any positive integer n , (x + y)n = n ∑ k = 0(n k)xn − kyk where (n k) = …
WebMar 31, 2024 · Transcript. Prove binomial theorem by mathematical induction. i.e. Prove that by mathematical induction, (a + b)^n = 𝐶(𝑛,𝑟) 𝑎^(𝑛−𝑟) 𝑏^𝑟 for any positive integer n, where C(n,r) = 𝑛!(𝑛−𝑟)!/𝑟!, n > r We need to prove (a + b)n = ∑_(𝑟=0)^𝑛 〖𝐶(𝑛,𝑟) 𝑎^(𝑛−𝑟) 𝑏^𝑟 〗 i.e. (a + b)n = ∑_(𝑟=0)^𝑛 … WebUse the Binomial Theorem to nd the expansion of (a+ b)n for speci ed a;band n. Use the Binomial Theorem directly to prove certain types of identities. ... The alternative to a …
WebNov 3, 2016 · We know that the binomial theorem and expansion extends to powers which are non-integers. For integer powers the expansion can be proven easily as the expansion is finite. However what is the proof that the expansion also holds for fractional powers? A simple an intuitive approach would be appreciated. binomial-coefficients binomial …
WebSep 10, 2024 · Binomial Theorem: Proof by Mathematical Induction This powerful technique from number theory applied to the Binomial Theorem Mathematical Induction is a proof technique that allows us... sims 4 put in inventory shortcutWebDec 21, 2024 · The expressions on the right-hand side are known as binomial expansions and the coefficients are known as binomial coefficients. More generally, for any nonnegative integer r, the binomial coefficient of xn in the binomial expansion of (1 + x)r is given by (rn) = r! n!(r − n)! and rcfhgWebMay 2, 2024 · It requires prior knowledge of combinations, mathematical induction. This expansion gives the formula for the powers of the binomial expression. Binomial expansion formula finds the expansion of powers of binomial expression very easily. ... Proof of binomial expansion using the principle of mathematical induction on n. Let … rcf hdl 26 aWeb5.2.2 Binomial theorem for positive integral index Now we prove the most celebrated theorem called Binomial Theorem. Theorem 5.1 (Binomial theorem for positive integral index): If nis any positive integer, then (a+b)n = nC 0 a b 0 + nC 1 a n−1b1 +···+ C ra n−rbr +···+ nC na 0bn. Proof. We prove the theorem by using mathematical induction. sims 4 pyxis ccWebWe can also use the binomial theorem directly to show simple formulas (that at first glance look like they would require an induction to prove): for example, 2 n= (1+1) = P n r=0. … sims 4 pyramid head ccWebStep 1. We have a binomial raised to the power of 4 and so we look at the 4th row of the Pascal’s triangle to find the 5 coefficients of 1, 4, 6, 4 and 1. Step 2. We start with (2𝑥) 4. It … rcf hdl 6 usateWebUse the Binomial Theorem to nd the expansion of (a+ b)n for speci ed a;band n. Use the Binomial Theorem directly to prove certain types of identities. ... The alternative to a combinatorial proof of the theorem is a proof by mathematical induction, which can be found following the examples illustrating uses of the theorem. Example 3: We start ... rcf hdl 6a buy in italy